Contents
Particles
Density
The density of a substance is defined as its mass per unit volume. If two objects are the same size but have different densities, the more dense object will be heavier.
Density can be calculated using the following equation (you DO need to remember this equation for your exam):
ρ = m ÷ v
ρ = density (kg/m3)
m = mass (kg)
v = volume (m3)
Worked Example
A wooden post has a volume of 0.025m3 and a mass of 20kg. Calculate its density.
ρ = m ÷ v
ρ = 20 ÷ 0.025
ρ = 800kg/m3
Density can have units of kg/m3 or g/cm3.
Converting units:
- 1 kg = 1000g
- 1m = 100cm
- 1m3 = 1,000,000 cm3
- 1000 kg/m3 = 1,000,000g ÷ 1,000,000cm3 = 1g/cm3
States of Matter
There are 3 states of matter:
- Solid
- Liquid
- Gas
They are defined by the arrangement and movement of their particles.
Solids
- Particles tightly packed together
- Vibrate in fixed positions
- Fixed shape
- Fixed volume (cannot be compressed)
- High density
Liquids
- Particles close together
- Move around randomly
- No fixed shape
- Fixed volume (cannot be compressed)
Gases
- Particles move around randomly at high speeds
- Particles far apart
- No fixed shape
- No fixed volume (can be compressed)
- Low density
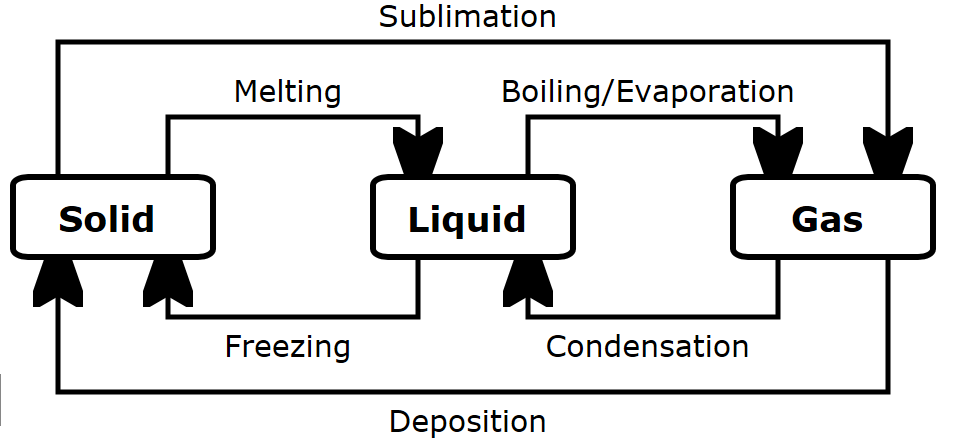
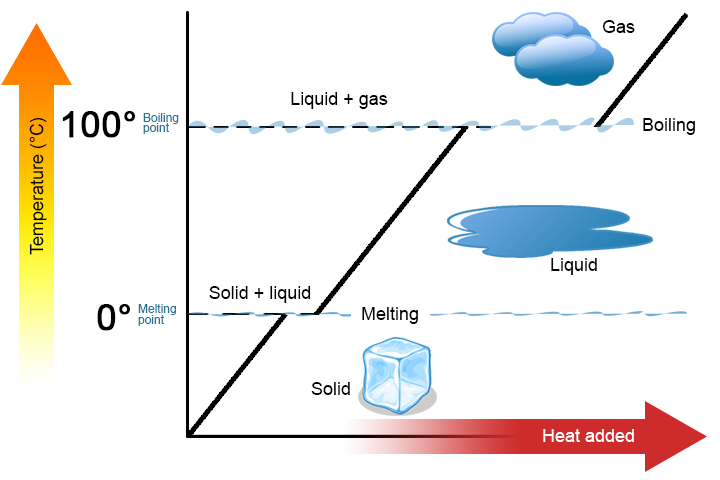
Changes of State
When a substance changes state, energy is transferred to or from the substance. Thermal energy is transferred to a solid to cause it to melt. Thermal energy is transferred from a liquid, causing it to freeze.
When a substance changes state, the number of particles remains the same. Therefore, the mass of the substance remains the same. This is known as conservation of mass.
As a substance is heated, its particles gain kinetic energy, allowing them to break free of their positions and melt or evaporate/boil. As a substance cools, its particles lose kinetic energy.
As a substance changes state, its temperature remains constant even though thermal energy is being transferred to it. This energy is called latent heat.
Internal Energy
The energy stored in the particles of a substance is called internal energy. Increasing the temperature of a substance increases its internal energy.
The internal energy depends on:
- The kinetic energy of each particle
- The potential energy of the particles based on the distance between them
- Video 1
- Video 2
Internal Energy in solids, liquids, and gases:
Solids
- Strong forces of attraction between particles
- Particles vibrate in fixed positions
- When heated, particles vibrate with more kinetic energy
- If heated enough, the solid melts – the particles break free of their positions
Liquids
- Weaker forces of attraction between particles – enough to keep them together, but not enough to keep them in fixed positions
- When heated, particles move faster
- If heated enough, the particles gain enough energy to break away from the other particles, becoming a gas
Gases
- Insignificant forces of attraction between particles
- Particles move around randomly at high speeds, colliding with each other and the sides of the container
- When heated, the particles gain kinetic energy and move faster
- They collide more often and with more force, increasing pressure
Latent Heat
When an object is changing state, its temperature does not increase even though it is being heated/cooled. Latent heat is the energy needed for a substance to change state.
- Specific Latent Heat of fusion is the amount of energy required to change 1kg of the substance from solid to liquid
- Specific Latent Heat of vaporisation is the amount of energy required to change 1kg of the substance from liquid to gas
Specific Latent heat can be calculated using this equation (you do NOT need to remember this equation for your exam):
- L = Specific Latent Heat (J/kg)
- E = Energy (J)
- m = mass (kg)
Worked Example
Calculate the mass of ice lost from an iceberg melting if the energy supplied was 835,000,000J.
Latent heat of fusion = 334,000 J/Kg
E = mL
m = E ÷ L
m = 835,000,000 ÷ 334,000
m = 2500kg
Measuring the specific latent heat of fusion of ice:
- Place ice in a funnel and collect the melted ice (water) that flows through the funnel in a measuring cylinder.
- Measure the mass of water collected in 10 minutes.
- Repeat but add an immersion heater to the ice.
- Measure the mass of water collected in 10 minutes with the heater on.
- The difference between those two masses is used as m in the equation.
- Calculate the specific latent heat of fusion using the equation.
Measuring the specific latent heat of vaporisation of water:
- Heat water to boiling point on an electronic balance.
- The mass change on the balance as the water evaporates is used as m in the equation.
Required Practical – Measuring Density
To calculate density for a regular shaped solid:
- Measure mass on a balance
- Calculate volume mathematically (length x width x height)
- Calculate density = mass ÷ volume
To calculate density for an irregular solid:
- Measure mass on a balance
- Measure volume using displacement – place the object in water and measure the amount of water displaced
- Calculate density = mass ÷ volume
To calculate density for a liquid:
- Measure mass on a balance (total mass – mass of container)
- Measure volume using a measuring cylinder
- Calculate density = mass ÷ volume
Possible causes of error in these investigations include:
- Inaccurate measurement of mass
- Inaccurate measurement of length, etc., of a regular shaped solid
- Inaccurate measurement of displaced water
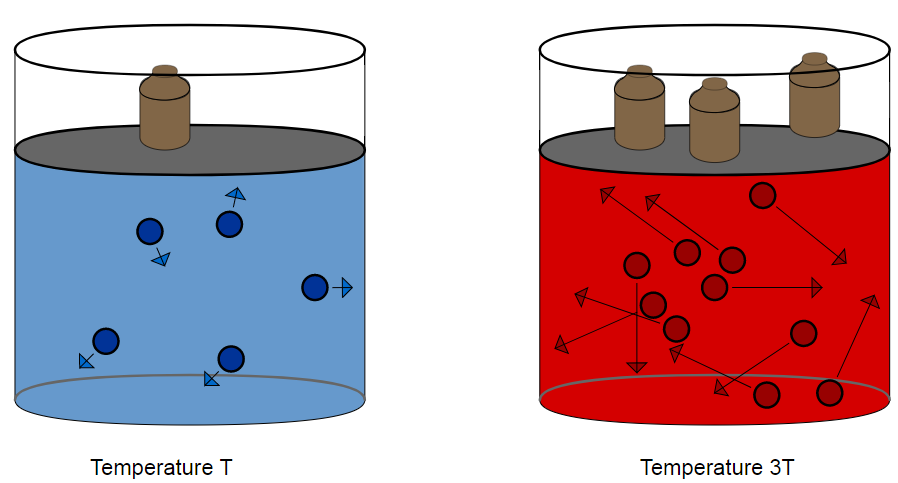
Gas Pressure
Brownian Motion
Individual molecules are too small to see directly, but their motion can be observed by their effect on other particles. Particles in a gas move randomly. They move very fast. They collide with each other and the sides of the container. This is called Brownian motion.
Gas Pressure and Temperature
Increasing the temperature of a gas will increase the pressure as the particles move faster, causing more frequent collisions. This means that the pressure will increase (if the volume remains constant).
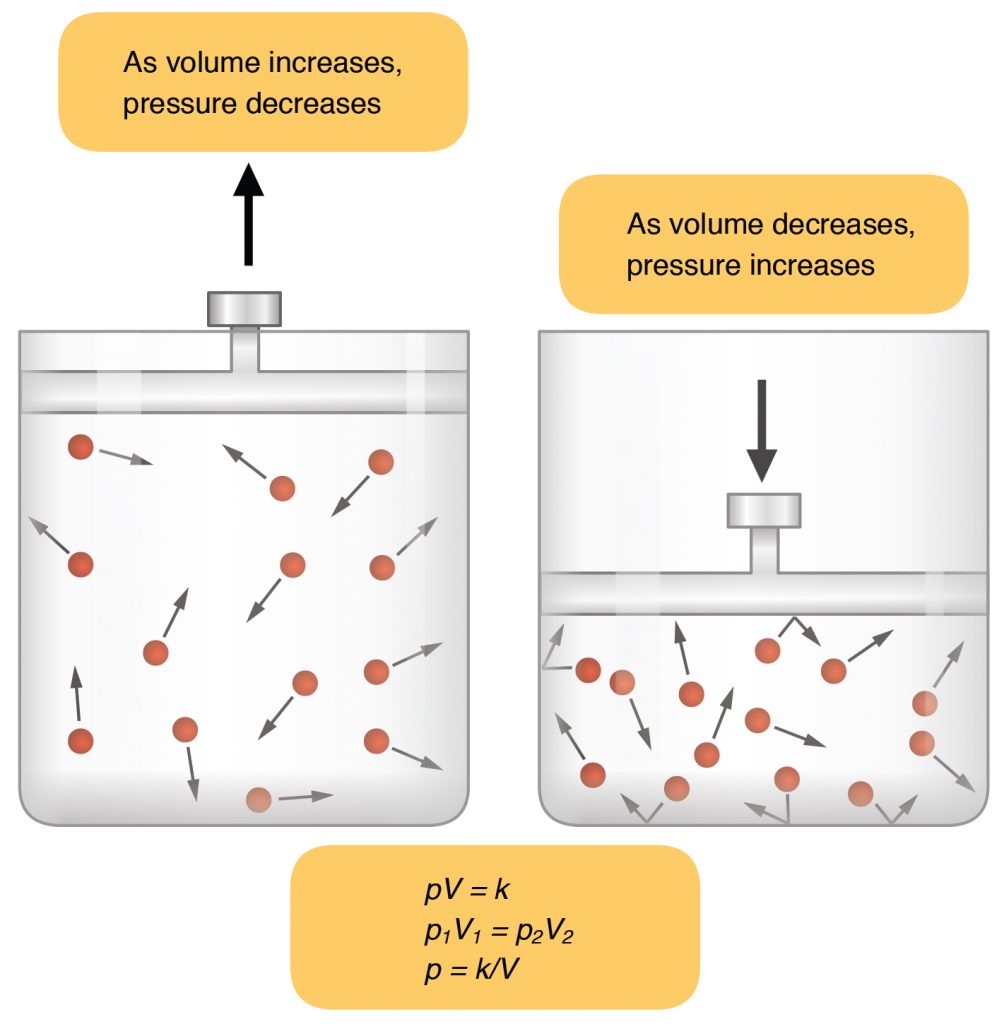
Pressure and Volume
The same amount of air molecules in a smaller volume will exert more pressure as there will be more collisions:
Boyle’s Law
Gas pressure is inversely proportional to volume
P x V = constant
P1V1 = P2V2
(you do NOT need to remember this equation for your exam)
- What is the density of an object with a mass of 45kg and a volume of 5m3?
- 9
- What is the mass of an object with a volume of 4m3 and a density of 5450kg/m3?
- 21800
- What is the volume of an object with a mass of 420kg and a density of 140kg/m3?
- 3
- A jeweler melts 0.5kg of gold to pour into a mold. Calculate how much energy was released as the gold solidified. (Latent heat of fusion of gold = 88000J/kg)
- 44000
- A kettle fails to switch off and boils for 5 minutes, wasting 600,000 J of energy. Calculate the mass of water lost during this time. (Latent heat of vaporisation of water = 2,260,000J/kg)
- 0.265